But the equation for a parabola can also be written in "vertex form" y = a ( x − h) 2 k In this equation, the vertex of the parabola is the point ( h, k) You can see how this relates to the standard equation by multiplying it out y = a ( x − h) ( x − h) k y = a x 2 − 2 a h x a h 2 k This means that in the standard form, yGraph y=x^22x8 y = x2 − 2x − 8 y = x 2 2 x 8 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for x 2 − 2 x − 8 x 2 2 x 8 Tap for more steps Use the form a x 2 b x c a x 2 b x c, to find the values of a a, b b, and c cDivide 2, the coefficient of the x term, by 2 to get 1 Then add the square of 1 to both sides of the equation This step makes the left hand side of the equation a perfect square Add y3 to 1 Factor x^ {2}2x1 In general, when x^ {2}bxc is a perfect square, it can always be factored as \left (x\frac {b} {2}\right)^ {2}

Y X
Y=x^2-2x+8 vertex form
Y=x^2-2x+8 vertex form-Standard form quadratic equation in vertex form Converting by Finding the Vertex 1 Find the xvalue of the vertex by using 2 Input the xvalue of the vertex back into the quadratic to find the yvalue of the vertex 3 Input the vertex into the h and k values of the vertex form, which is y = a(x Convert to vertex form by completing the square 1 y = x 2 4x 2 y = x 2 2x 5 3 y = x 2 14x 59 4 y = 2x 2 36x 170
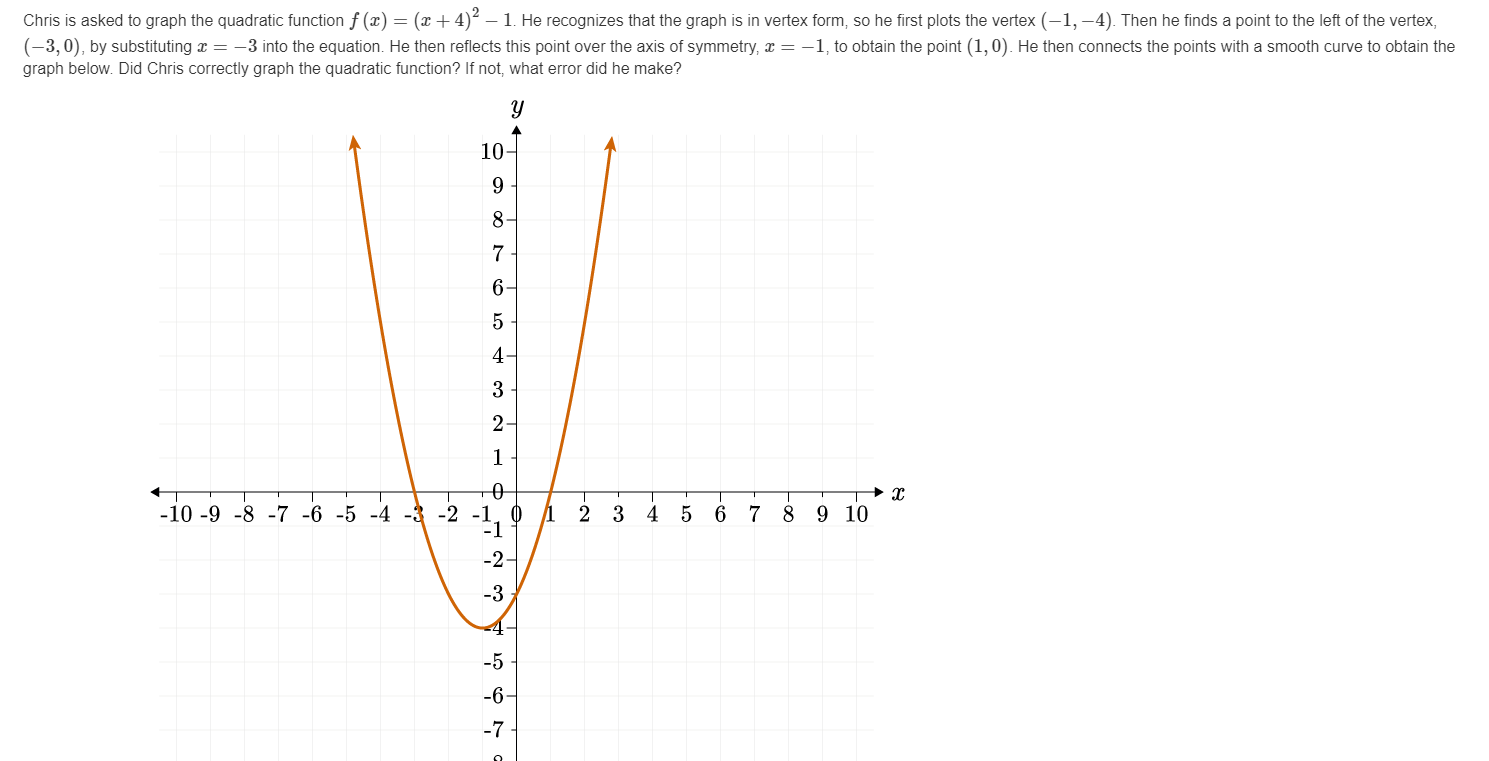



Chris Is Asked To Graph The Quadratic Function F X Chegg Com
How do I Change y=x^22x8 into factored form and then use the zero product property to find the x intercepts? To change the expression (x2 2x) into a perfect square trinomial add (half the x coefficient)² to each side of the expression Here x coefficient = 2 So, (half the x coefficient)2 = (2/2)2 = 1 Add and subtract 1 to the expression The vertex form of the parabola with vertex (h, k) and axis of symmetry x = h is y = a (x h)2 kFind the Vertex Form y=x^26x8 Complete the square for Tap for more steps Use the form , to find the values of , , and Consider the vertex form of a parabola Substitute the values of and into the formula Simplify the right side Tap for more steps Cancel the common factor of and
Solved by pluggable solver Completing the Square to Get a Quadratic into Vertex Form Start with the given equation Subtract from both sides Factor out the leading coefficient Take half of the x coefficient to get (ie ) Now square to get (ie ) Now add andHow do I find the vertex and the equation of the line of symmetry and graph?Divide 22\sqrt {y} by 2 The equation is now solved Swap sides so that all variable terms are on the left hand side Factor x^ {2}2x1 In general, when x^ {2}bxc is a perfect square, it can always be factored as \left (x\frac {b} {2}\right)^ {2} Take the square root of
Vertex Form Equation & Functions Just as y = mx b is a useful format for graphing linear functions, y = a(x h)^2 k is a useful format for graphing quadratic functionsThe vertex has an xvalue of b/2a=(16)/2=8 f(8)= 1 Therefore, the vertex is at (8,1) The vertex form is (xh)^2 k, where you change the sign of the x value of the vertex (h) and keep the y component (k) y=(x8)^2 1 Expand that for x^216x641 x^216x63Give the vertex of each function, and graph it How does vertex form compare to the other forms in each problem?




17 Transform Quadratic Functi See How To Solve It At Qanda



Axis Of Symmetry
Question For the quadratic function f(x) = x^2 2x 8 Find a THe axis of symmetry and the vertex b THe xintercepts and y intercepts c The domain and range d Does the function have a maximum or minimum valueSolve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreParabola, Finding the Vertex 21 Find the Vertex of y = x 22x8 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting "y" because the coefficient of the first term, 1 , is positive (greater than zero)



Biomath Quadratic Functions




Chapter 5 Study Guide
The equation in vertex form is , y=(x4)^21 The equation in vertex form is, y=x^28x15= (x4)^ =(x4)^21 Vertex is at (4,1) graph{x^28x15 10, 10,What Is The Axis Of Symmetry And Vertex For The Graph F X X 2 2x 8 Socratic For more information and source, Solution 3 The Graph Of Y X 2 2x 8 Intersects The X Axis At 1 2 And 4 2 2 And 4 3 2 And 4 4 2 And 4 Please Show Steps For more information and source,Learn to complete the square in order to write quadratic equations in vertex form Click Create Assignment to assign this modality to your LMS We have a new and improved read on this topic



Www Scasd Org Cms Lib Pa Centricity Domain 1229 2 2 a2 17 unit 2 2 ws packet lg Pdf




Exercises 2 3 In Problems 1 8 Find The Vertex Form Of Chegg Com
Problem y= x^24x6Complete the square with the x terms x^24x4 = y64 (x2)^2 = y2 ===== This shows you the vertex is at (2,2) and p= 1/4 ===== Cheers, Stan H Answer by jim_thompson5910() (ShowAnswer to Find the root of an equation y=x^22x8 By signing up, you'll get thousands of stepbystep solutions to your homework questionsDivide 0 0 by − 8 8 Multiply − 1 1 by 0 0 Add 8 8 and 0 0 Substitute the values of a a, d d, and e e into the vertex form a ( x d) 2 e a ( x d) 2 e Set y y equal to the new right side Use the vertex form, y = a(x−h)2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k k



Practice 1 2 Graphing In Vertex Form And Intercept Form




Quadratic Equations And Quadratic Functions On The Sat
A) y = 2x 2 12x 16 B) y = 2x (x 6) 16 C) y = 2 (x 3) 2 (2) D) y = (x 2) (2x 8) Answer If you know the vertex form of an equation y = a (x h) 2 k then you will notice that only answer C is in that form Go to next Question Try the free Mathway calculator and problem solver below to practice various math topicsThe vertex form is a special form of a quadratic function From the vertex form, it is easily visible where the maximum or minimum point (the vertex) of the parabola is The number in brackets gives (trouble spot up to the sign!) the xcoordinate of the vertex, the number at the end of the form gives the ycoordinate36 is the value for 'c' that we found to make the right hand side a perfect square trinomial;




Ppt Building Understanding For Quadratics Powerpoint Presentation Free Download Id



Http Www Manhassetschools Org Cms Lib8 Ny Centricity Domain 710 Group work 59 63 solutions Pdf


