EduRev JEE Question is disucussed on EduRev Study Group by 185 JEE StudentsCancel (x 1) length = 2x 3 units The synthetic division method is in the attached pictureThe area of the region between the curves is defined as the integral of the upper curve minus the integral of the lower curve over each region The regions are determined by the intersection points of the curves This can be done algebraically or graphically Integrate to find the area between and
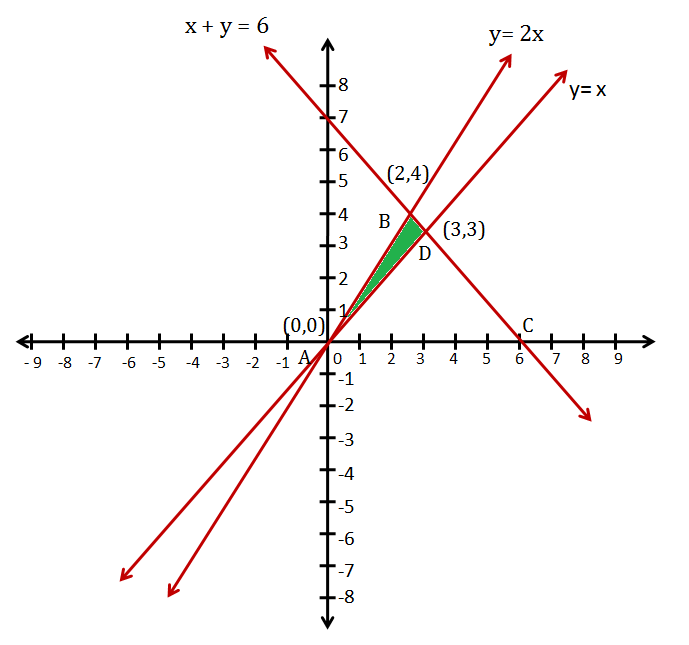
Determine Graphically The Co Ordinates Of The Vertices Of The Triangle Formed By The Equation Whose Sides Are Y X Y 2x X Y 6 Also Area And Co Ordinate Mathematics Topperlearning Com Ee7ugaa
2x y=2 2y-x=4. what is the area of the triangle formed by these lines and the x-axis
2x y=2 2y-x=4. what is the area of the triangle formed by these lines and the x-axis-In graph we find that the lines are intersecting at (1, 4) Hence x = 1, y = 4 is the solution of the equationsThe two triangles are ABC and DBE Area of ABE = × Base × perpendicular Area of ADC = × Base × perpendicular ABE area ADC Area The triangular region bounded by the lines y=2x1, y=3x1 and x=4 is represented graphically as Equations of the lines are y=2x1,y=3x1 and x4 Let y 1 =2x 1, y 2 = 3x1 Now area of the triangle bounded by the given lines = 8 square units Thus, the area of the required triangular region is 8 sq units
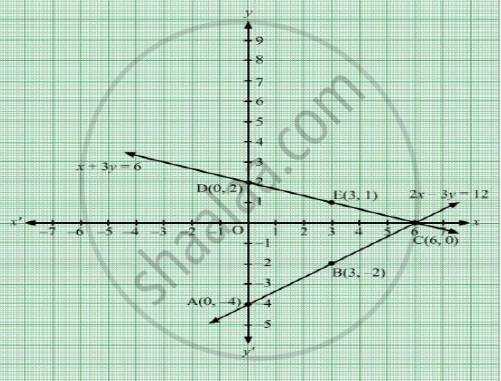



Solve Graphically The System Of Equations 2x 3y 12 X 3y 6 Find The Coordinates Of The Vertices Of The Triangle Formed By These Two Lines And The Y Axis Mathematics Shaalaa Com
A line that runs parallel to the \(x\)axis is called a horizontal line and has a gradient of zero This is because there is no vertical change \m = \frac{\text{change in } y}{\text{change in } x} = \frac{0}{\text{change in } x} = 0\ A line that runs parallel to the \(y\)axis is called a vertical line and its gradient is undefined What is the area of triangle formed by line 2x3y=12 with the coordinate axes?Solution Question 39 Draw the graphs of the following equations x y — 5;
The zeros are 4 and 12 Function 2 and 3 intersect in P (0,4) 61E Exercises for Section 61 For exercises 1 2, determine the area of the region between the two curves in the given figure by integrating over the xaxis For exercises 3 4, split the region between the two curves into two smaller regions, then determine the area by integrating over the xOf the tetrahedron The base is the triangle in the xyplane sketched below What's the equation for the line?
In Geometry, a triangle is a threesided polygon that has three edges and three vertices The area of the triangle is the space covered by the triangle in a twodimensional plane The formula for the area of a triangle is (1/2) × base × altitude Let's find out the area of a triangleThe area of the triangle formed by the lines 3x 2y = 8, 2x y = 1 and the xaxis has to be determined First, determine the point of intersection of the lines 3x 2y = 8 and 2x y = 1Solve the Following System of Equations Graphically 2x = 23 3y 5x = 8y Also, Find the Area of the Triangle Formed by These Lines and Xaxis in Each Graph



Class 10 Maths Graphical Questions For Linear Equations In Two Variables
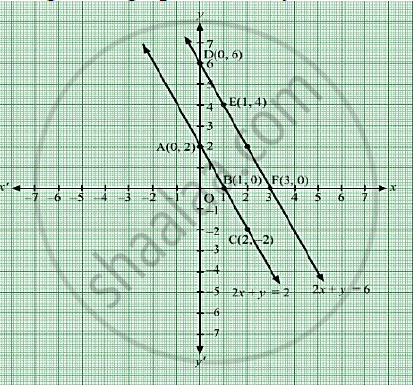



Draw Graphs For Following Equations On The Same Graph Paper 2x Y 2 2x Y 6 Find Co Ordinates Of The Vertices Of Trapezium Formed By These Lines Also
Question A triangle is formed by the intersection of the lines 2x 3y = 14 4X 5y= 16 and the x axisFind the area of the triangle Thank you so much Answer by ReadingBoosters(3246) (Show Source) Clearly, we can scale the coefficients of a given linear equation by any (nonzero) constant and the result is unchanged Therefore, by dividingthrough by $\sqrt{a_i^2b_i^2}$, we may assume our equations are in "normal form"The area of the triangle formed the lines x=3, y=4 and x=y is MCQ Chapter 3 RD Class 10 🔥🔥 The area of the triangle formed the lines x=3, y=4 and x=y is MCQ Chapter 3




Solve The Following Pair Of Equations Graphically X 3y 6 2x 3y 12 Also Find The Area Of The Brainly In




Solution Find The Angle Formed By The Lines 2x Y 8 0 And X
math 1)The area A of a triangle varies jointly as the lengths of its base b and height h If A is 75 when b=15 and h=10,find A when b=8 and h=6 2)Determine the equation of any vertical asymtotes of the graph of f (x)=2x3/x^22x3 1)X − 2 = 0 x 2 = 0 Add 2 2 to both sides of the equation x = 2 x = 2 x = 2 x = 2 The final solution is all the values that make x ( x − 2) = 0 x ( x 2) = 0 true x = 0, 2 x = 0, 2 x = 0, 2 x = 0, 2 x = 0, 2 x = 0, 2 Substitute 0 0 for x x into y = 2 x y = 2 x then solve for y y Given 2xy=2 , 2yx=4 To Find the area of a triangle formed by the two lines and the line y=0 Solution 2xy=2 2yx=4 y = 0 2xy=2 , y = 0 => point of intersection ( 1 , 0) 2yx=4 , y = 0 => point of intersection ( 4 , 0) 2xy=2 , 2yx=4 => point of intersection ( 0 , 2) Triangle is formed with base = ( 1 (4) = 5 Height = 2
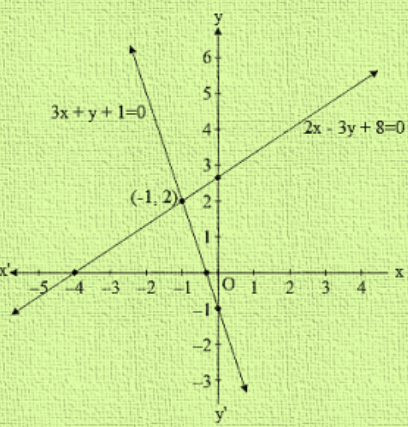



R D Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2




Determine Graphically The Co Ordinates Of The Vertices Of The Triangle Formed By The Equation Whose Sides Are Y X Y 2x X Y 6 Also Area And Co Ordinate Mathematics Topperlearning Com Ee7ugaa
🔴 Answer 3 🔴 on a question The area of a triangle is 1/2 (x^2 2x 2x^2 4) the height h is x2 write an expression for the base b of the triangle ( hint area of a triangle 1/2 bh) the answers to ihomeworkhelperscom Given 2xy=2 , 2yx=4 To Find the area of a triangle formed by the two lines and the line y=0 Solution 2xy=2 2yx=4 y = 0 2xy=2 , y = 0 => point of intersection ( 1 , 0) 2yx=4 , y = 0 => point of intersection ( 4 , 0) 2xy=2 , 2yx=4 => point of intersection ( 0 , 2) Triangle is formed with base = ( 1 (4) = 5 Height = 2 asked in Class X Maths by saurav24 Expert (14k points) Draw the graph of the pair of equations 2x y= 4 and 2x – y = 4 Write the vertices of the triangle formed by these lines and the yaxis, find the area of this triangle?



What Is The Area Of The Triangle Formed By 2x 3y 6 And The Coordinate Axes Quora




Solve The Equations Graphically 2x Y 2 2y X 4 Also Find The Area Of A Triangle Fo Brainly In
Xy = 5 (i) Find the solution of the equations from the graph (ii) Shade the triangular region formed by the lines and theyaxis Solution 10Answer 2xy = 4(i) ⇒ y = 4−2x If x= 0,y = 4−2(0)= 4−0 =4 x = 1,y = 4−2(1) = 4−2= 2 x = 2,y = 4−2(2) = 4−4= 0 x 0 1 2 3 y 4 2 0 −2 (i) A B C −2If 2x^23xyy^2=0 represents two sides of a triangle and lxmyn=0 in the third side then locus of incentre of the triangle is




R D Sharma Class 10 Solutions Maths Chapter 3 Pair Of Linear Equations In Two Variables Exercise 3 2
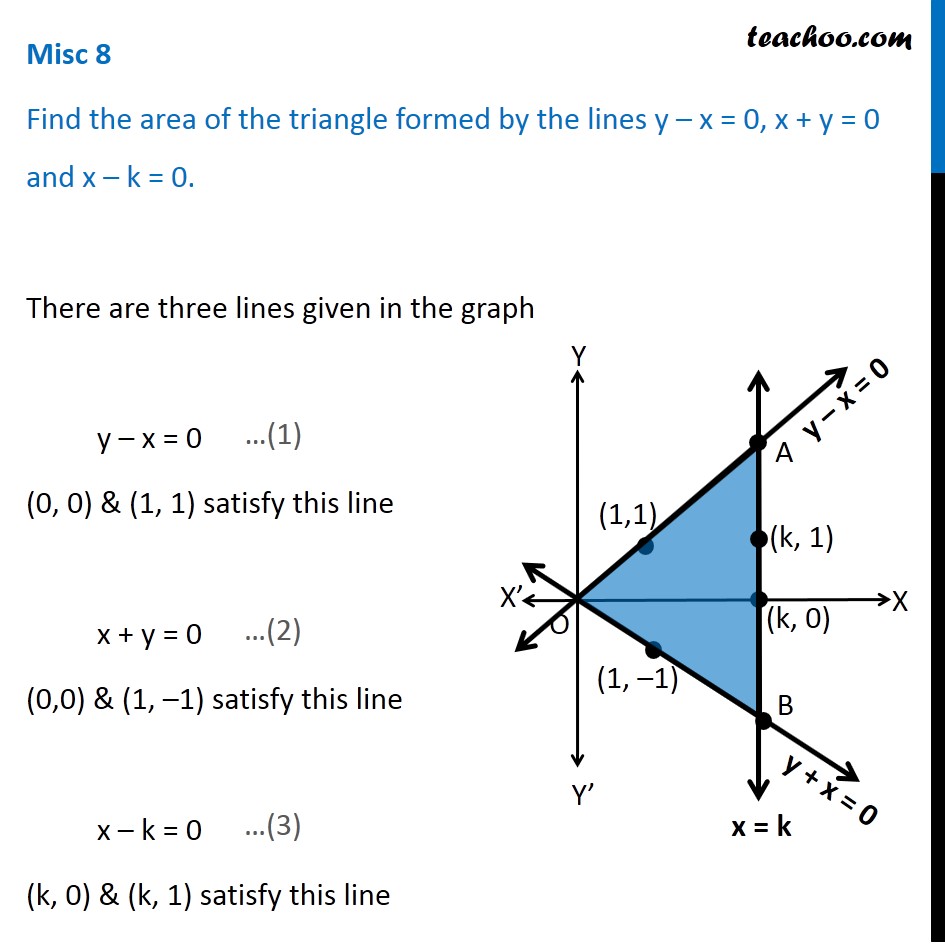



Misc 8 Find Area Of Triangle Formed By Y X 0 X Y 0


